Phase transitions are processes that convert matter from one physical state into another. The temperatures at which phase transitions occur are determined by the relative strengths of intermolecular attractions and are, therefore, dependent on the chemical identity of the substance. Boiling point elevation and freezing point depression are examples of colligative properties that occur due to the addition of a non-volatile solute to a volatile solvent.
5.1 Phase Transitions
By the end of this section, you will be able to:
- Define phase transitions and phase transition temperatures
- Explain the relation between phase transition temperatures and intermolecular attractive forces
- Describe the processes represented by typical heating and cooling curves, and compute heat flows and enthalpy changes accompanying these processes
We witness and utilize changes of physical state, or phase transitions, in a great number of ways. As one example of global significance, consider the evaporation, condensation, freezing, and melting of water. These changes of state are essential aspects of our earth’s water cycle as well as many other natural phenomena and technological processes of central importance to our lives. In this module, the essential aspects of phase transitions are explored.
5.1.1 Boiling Points
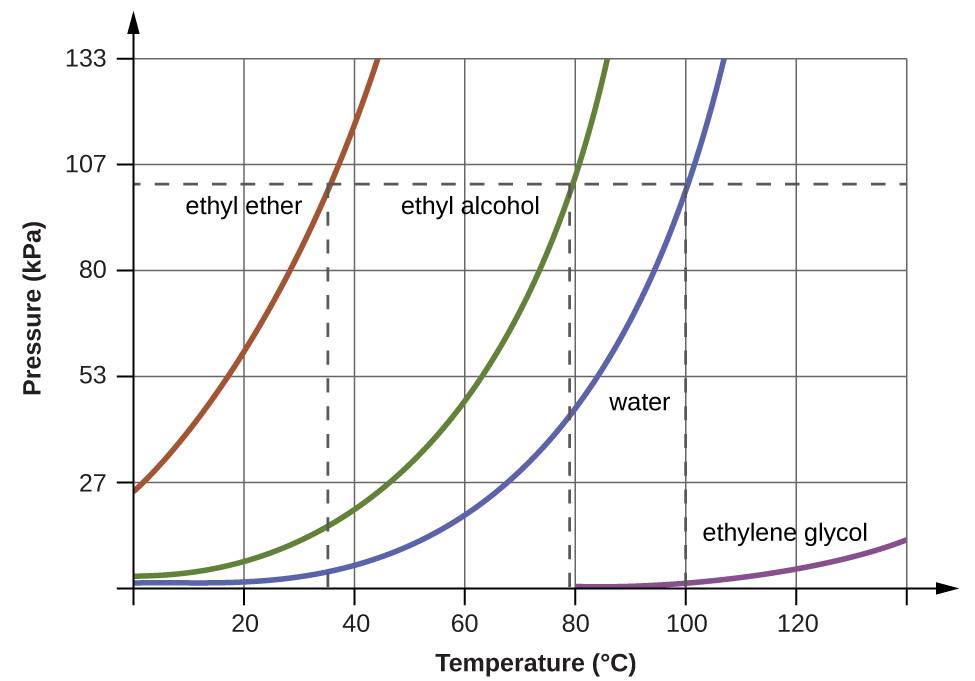
When the vapor pressure increases enough to equal the external atmospheric pressure, the liquid reaches its boiling point. The boiling point of a liquid is the temperature at which its equilibrium vapor pressure is equal to the pressure exerted on the liquid by its gaseous surroundings. For liquids in open containers, this pressure is that due to the earth’s atmosphere. The normal boiling point of a liquid is defined as its boiling point when surrounding pressure is equal to 1 atm (101.3 kPa). Figure 5.1 shows the variation in vapor pressure with temperature for several different substances. Considering the definition of boiling point, these curves may be seen as depicting the dependence of a liquid’s boiling point on surrounding pressure.
Figure 5.1
The boiling points of liquids are the temperatures at which their equilibrium vapor pressures equal the pressure of the surrounding atmosphere. Normal boiling points are those corresponding to a pressure of 1 atm (101.3 kPa.)
A Boiling Point at Reduced Pressure
A typical atmospheric pressure in Leadville, Colorado (elevation 10,200 feet) is 68 kPa. Use the graph in
Figure 5.1 to determine the boiling point of water at this elevation.
Solution
The graph of the vapor pressure of water versus temperature in
Figure 5.1 indicates that the vapor pressure of water is 68 kPa at about 90 °C. Thus, at about 90 °C, the vapor pressure of water will equal the atmospheric pressure in Leadville, and water will boil.
Check Your Learning
The boiling point of ethyl ether was measured to be 10 °C at a base camp on the slopes of Mount Everest. Use figure
Figure5.1 to determine the approximate atmospheric pressure at the camp.
Approximately 40 kPa (0.4 atm)
5.1.3 Melting and Freezing
When we heat a crystalline solid, we increase the average energy of its atoms, molecules, or ions and the solid gets hotter. At some point, the added energy becomes large enough to partially overcome the forces holding the molecules or ions of the solid in their fixed positions, and the solid begins the process of transitioning to the liquid state, or melting. At this point, the temperature of the solid stops rising, despite the continual input of heat, and it remains constant until all of the solid is melted. Only after all of the solid has melted will continued heating increase the temperature of the liquid (Figure 5.2).
Figure 5.2
(a) This beaker of ice has a temperature of −12.0 °C. (b) After 10 minutes the ice has absorbed enough heat from the air to warm to 0 °C. A small amount has melted. (c) Thirty minutes later, the ice has absorbed more heat, but its temperature is still 0 °C. The ice melts without changing its temperature. (d) Only after all the ice has melted does the heat absorbed cause the temperature to increase to 22.2 °C. (credit: modification of work by Mark Ott)

If we stop heating during melting and place the mixture of solid and liquid in a perfectly insulated container so no heat can enter or escape, the solid and liquid phases remain in equilibrium. This is almost the situation with a mixture of ice and water in a very good thermos bottle; almost no heat gets in or out, and the mixture of solid ice and liquid water remains for hours. In a mixture of solid and liquid at equilibrium, the reciprocal processes of melting and freezing occur at equal rates, and the quantities of solid and liquid therefore remain constant. The temperature at which the solid and liquid phases of a given substance are in equilibrium is called the melting point of the solid or the freezing point of the liquid. Use of one term or the other is normally dictated by the direction of the phase transition being considered, for example, solid to liquid (melting) or liquid to solid (freezing).
The enthalpy of fusion and the melting point of a crystalline solid depend on the strength of the attractive forces between the units present in the crystal. Molecules with weak attractive forces form crystals with low melting points. Crystals consisting of particles with stronger attractive forces melt at higher temperatures.
The amount of heat required to change one mole of a substance from the solid state to the liquid state is the enthalpy of fusion, ΔHfus of the substance. The enthalpy of fusion of ice is 6.0 kJ/mol at 0 °C. Fusion (melting) is an endothermic process:
The reciprocal process, freezing, is an exothermic process whose enthalpy change is −6.0 kJ/mol at 0 °C:
5.1.4 Boiling Point Elevation
As described in the chapter on liquids and solids, the boiling point of a liquid is the temperature at which its vapor pressure is equal to ambient atmospheric pressure. Since the vapor pressure of a solution is lowered due to the presence of nonvolatile solutes, it stands to reason that the solution’s boiling point will subsequently be increased. Vapor pressure increases with temperature, and so a solution will require a higher temperature than will pure solvent to achieve any given vapor pressure, including one equivalent to that of the surrounding atmosphere. The increase in boiling point observed when nonvolatile solute is dissolved in a solvent, ΔTb, is called boiling point elevation and is directly proportional to the molal concentration of solute species:
where Kb is the boiling point elevation constant, or the ebullioscopic constant and m is the molal concentration (molality) of all solute species.
Boiling point elevation constants are characteristic properties that depend on the identity of the solvent. Values of Kb for several solvents are listed in Table 5.1.
Table 5.3
Boiling Point Elevation and Freezing Point Depression Constants for Several Solvents
| Solvent | Boiling Point (°C at 1 atm) | Kb (ºCm−1) | Freezing Point (°C at 1 atm) | Kf (ºCm−1) |
|---|
| water | 100.0 | 0.512 | 0.0 | 1.86 |
| hydrogen acetate | 118.1 | 3.07 | 16.6 | 3.9 |
| benzene | 80.1 | 2.53 | 5.5 | 5.12 |
| chloroform | 61.26 | 3.63 | −63.5 | 4.68 |
| nitrobenzene | 210.9 | 5.24 | 5.67 | 8.1 |
The extent to which the vapor pressure of a solvent is lowered and the boiling point is elevated depends on the total number of solute particles present in a given amount of solvent, not on the mass or size or chemical identities of the particles. A 1 m aqueous solution of sucrose (342 g/mol) and a 1 m aqueous solution of ethylene glycol (62 g/mol) will exhibit the same boiling point because each solution has one mole of solute particles (molecules) per kilogram of solvent.
Calculating the Boiling Point of a Solution
Assuming ideal solution behavior, what is the boiling point of a 0.33
m solution of a nonvolatile solute in benzene?
Solution
Use the equation relating boiling point elevation to solute molality to solve this problem in two steps.

- Step 1. Calculate the change in boiling point.
- Step 2. Add the boiling point elevation to the pure solvent’s boiling point.
Check Your Learning
Assuming ideal solution behavior, what is the boiling point of the antifreeze described in
Example 11.3?
The Boiling Point of an Iodine Solution
Find the boiling point of a solution of 92.1 g of iodine, I
2, in 800.0 g of chloroform, CHCl
3, assuming that the iodine is nonvolatile and that the solution is ideal.
Solution
A four-step approach to solving this problem is outlined below.
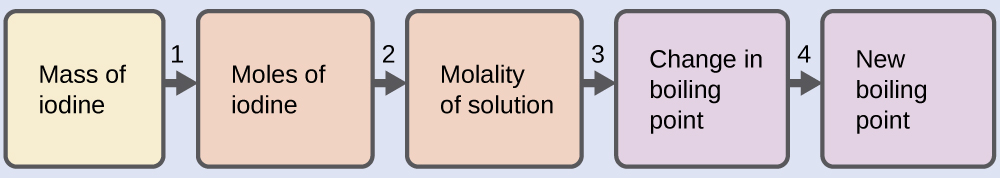
- Step 1. Convert from grams to moles of I2 using the molar mass of I2 in the unit conversion factor.
Result: 0.363 mol - Step 2. Determine the molality of the solution from the number of moles of solute and the mass of solvent, in kilograms.
Result: 0.454 m - Step 3. Use the direct proportionality between the change in boiling point and molal concentration to determine how much the boiling point changes.
Result: 1.65 °C - Step 4. Determine the new boiling point from the boiling point of the pure solvent and the change.
Result: 62.91 °C
Check each result as a self-assessment.
Check Your Learning
What is the boiling point of a solution of 1.0 g of glycerin, C
3H
5(OH)
3, in 47.8 g of water? Assume an ideal solution.
5.1.7 Freezing Point Depression
Solutions freeze at lower temperatures than pure liquids. This phenomenon is exploited in “de-icing” schemes that use salt (Figure 5.3), calcium chloride, or urea to melt ice on roads and sidewalks, and in the use of ethylene glycol as an “antifreeze” in automobile radiators. Seawater freezes at a lower temperature than fresh water, and so the Arctic and Antarctic oceans remain unfrozen even at temperatures below 0 °C (as do the body fluids of fish and other cold-blooded sea animals that live in these oceans).
Figure 5.4
Rock salt (NaCl), calcium chloride (CaCl2), or a mixture of the two are used to melt ice. (credit: modification of work by Eddie Welker)

The decrease in freezing point of a dilute solution compared to that of the pure solvent, ΔTf, is called the freezing point depression and is directly proportional to the molal concentration of the solute
where m is the molal concentration of the solute and Kf is called the freezing point depression constant (or cryoscopic constant). Just as for boiling point elevation constants, these are characteristic properties whose values depend on the chemical identity of the solvent. Values of Kf for several solvents are listed in Table 5.1.
Calculation of the Freezing Point of a Solution
Assuming ideal solution behavior, what is the freezing point of the 0.33
m solution of a nonvolatile nonelectrolyte solute in benzene described in
Example 11.4?
Solution
Use the equation relating freezing point depression to solute molality to solve this problem in two steps.

- Step 1. Calculate the change in freezing point.
- Step 2. Subtract the freezing point change observed from the pure solvent’s freezing point.
Check Your Learning
Assuming ideal solution behavior, what is the freezing point of a 1.85
m solution of a nonvolatile nonelectrolyte solute in nitrobenzene?
5.1.9 CHEMISTRY IN EVERYDAY LIFE
Colligative Properties and De-Icing
Sodium chloride and its group 2 analogs calcium and magnesium chloride are often used to de-ice roadways and sidewalks, due to the fact that a solution of any one of these salts will have a freezing point lower than 0 °C, the freezing point of pure water. The group 2 metal salts are frequently mixed with the cheaper and more readily available sodium chloride (“rock salt”) for use on roads, since they tend to be somewhat less corrosive than the NaCl, and they provide a larger depression of the freezing point, since they dissociate to yield three particles per formula unit, rather than two particles like the sodium chloride.
Because these ionic compounds tend to hasten the corrosion of metal, they would not be a wise choice to use in antifreeze for the radiator in your car or to de-ice a plane prior to takeoff. For these applications, covalent compounds, such as ethylene or propylene glycol, are often used. The glycols used in radiator fluid not only lower the freezing point of the liquid, but they elevate the boiling point, making the fluid useful in both winter and summer. Heated glycols are often sprayed onto the surface of airplanes prior to takeoff in inclement weather in the winter to remove ice that has already formed and prevent the formation of more ice, which would be particularly dangerous if formed on the control surfaces of the aircraft (Figure 5.4).
Figure 5.5
Freezing point depression is exploited to remove ice from (a) roadways and (b) the control surfaces of aircraft.

The colligative effects on vapor pressure, boiling point, and freezing point described in the previous section are conveniently summarized by comparing the phase diagrams for a pure liquid and a solution derived from that liquid (Figure 5.5).
Figure 5.6
Phase diagrams for a pure solvent (solid curves) and a solution formed by dissolving nonvolatile solute in the solvent (dashed curves).
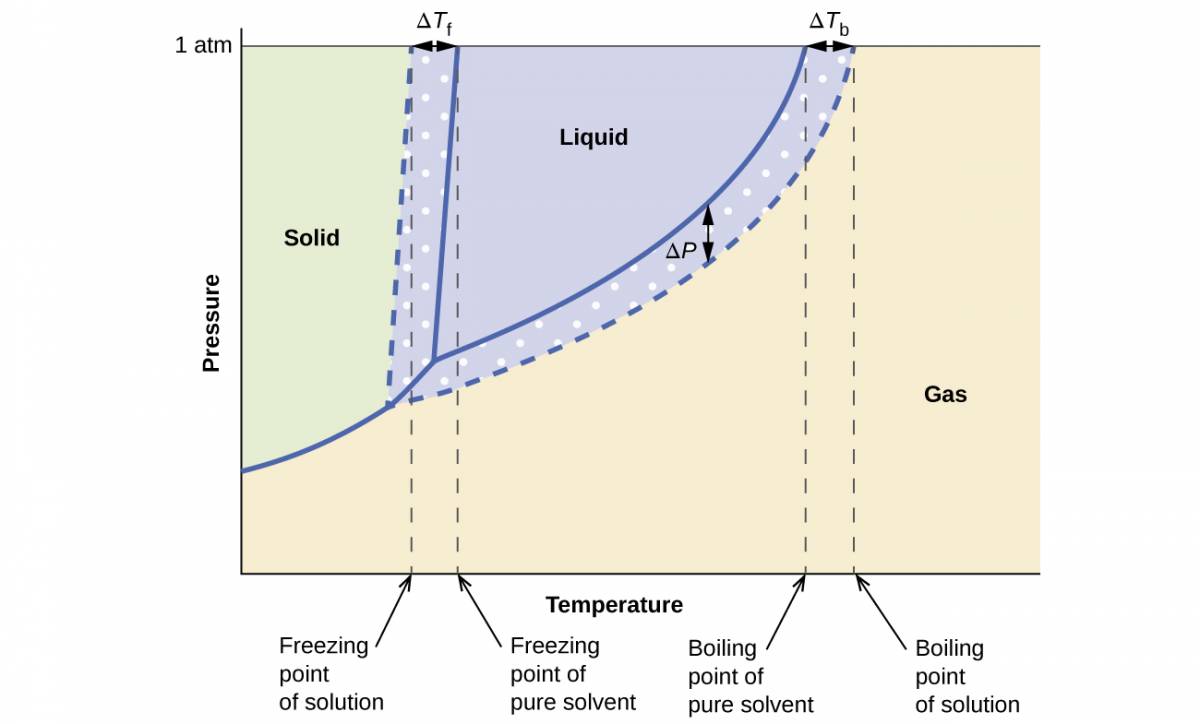
The liquid-vapor curve for the solution is located beneath the corresponding curve for the solvent, depicting the vapor pressure lowering, ΔP, that results from the dissolution of nonvolatile solute. Consequently, at any given pressure, the solution’s boiling point is observed at a higher temperature than that for the pure solvent, reflecting the boiling point elevation, ΔTb, associated with the presence of nonvolatile solute. The solid-liquid curve for the solution is displaced left of that for the pure solvent, representing the freezing point depression, ΔTf, that accompanies solution formation. Finally, notice that the solid-gas curves for the solvent and its solution are identical. This is the case for many solutions comprising liquid solvents and nonvolatile solutes. Just as for vaporization, when a solution of this sort is frozen, it is actually just the solvent molecules that undergo the liquid-to-solid transition, forming pure solid solvent that excludes solute species. The solid and gaseous phases, therefore, are composed of solvent only, and so transitions between these phases are not subject to colligative effects.
EXAMPLE 5.1.10
The Freezing Point of a Solution of an Electrolyte
The concentration of ions in seawater is approximately the same as that in a solution containing 4.2 g of NaCl dissolved in 125 g of water. Use this information and a predicted value for the van’t Hoff factor (Table 11.3) to determine the freezing temperature the solution (assume ideal solution behavior).
Solution
Solve this problem using the following series of steps.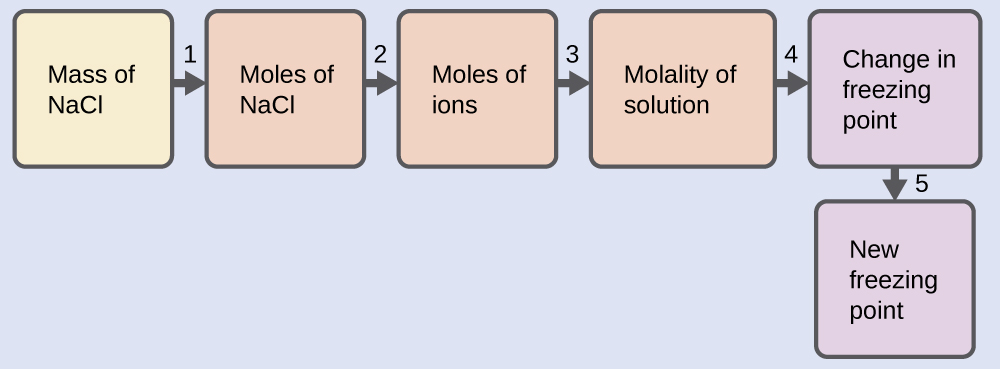
- Step 1. Convert from grams to moles of NaCl using the molar mass of NaCl in the unit conversion factor.
Result: 0.072 mol NaCl - Step 2. Determine the number of moles of ions present in the solution using the number of moles of ions in 1 mole of NaCl as the conversion factor (2 mol ions/1 mol NaCl).
Result: 0.14 mol ions - Step 3. Determine the molality of the ions in the solution from the number of moles of ions and the mass of solvent, in kilograms.
Result: 1.2 m - Step 4. Use the direct proportionality between the change in freezing point and molal concentration to determine how much the freezing point changes.
Result: 2.1 °C - Step 5. Determine the new freezing point from the freezing point of the pure solvent and the change.
Result: −2.1 °C
Check each result as a self-assessment, taking care to avoid rounding errors by retaining guard digits in each step’s result for computing the next step’s result.
Check Your Learning
Assuming complete dissociation and ideal solution behavior, calculate the freezing point of a solution of 0.724 g of CaCl2 in 175 g of water.