10.1 Reaction Mechanisms

Chemical reactions very often occur in a step-wise fashion, involving two or more distinct reactions taking place in sequence. A balanced equation indicates what is reacting and what is produced, but it reveals no details about how the reaction actually takes place. The reaction mechanism (or reaction path) provides details regarding the precise, step-by-step process by which a reaction occurs.

The decomposition of ozone, for example, appears to follow a mechanism with two steps:

Each of the steps in a reaction mechanism is an elementary reaction. These elementary reactions occur precisely as represented in the step equations, and they must sum to yield the balanced chemical equation representing the overall reaction:

Notice that the oxygen atom produced in the first step of this mechanism is consumed in the second step and therefore does not appear as a product in the overall reaction. Species that are produced in one step and consumed in a subsequent step are called intermediates.

While the overall reaction equation for the decomposition of ozone indicates that two molecules of ozone react to give three molecules of oxygen, the mechanism of the reaction does not involve the direct collision and reaction of two ozone molecules. Instead, one O₃ decomposes to yield O₂ and an oxygen atom, and a second O₃ molecule subsequently reacts with the oxygen atom to yield two additional O₂ molecules.

Unlike balanced equations representing an overall reaction, the equations for elementary reactions are explicit representations of the chemical change taking place. The reactant(s) in an elementary reaction’s equation undergo only the bond-breaking and/or making events depicted to yield the product(s). For this reason, the rate law for an elementary reaction may be derived directly from the balanced chemical equation describing the reaction. This is not the case for typical chemical reactions, for which rate laws may be reliably determined only via experimentation.

10.1.1 Unimolecular Elementary Reactions

The molecularity of an elementary reaction is the number of reactant species (atoms, molecules, or ions). For example, a unimolecular reaction involves the reaction of a single reactant species to produce one or more molecules of product:

The rate law for a unimolecular reaction is first order:

A unimolecular reaction may be one of several elementary reactions in a complex mechanism. For example, the reaction:

illustrates a unimolecular elementary reaction that occurs as one part of a two-step reaction mechanism as described above. However, some unimolecular reactions may be the only step of a single-step reaction mechanism. (In other words, an “overall” reaction may also be an elementary reaction in some cases.) For example, the gas-phase decomposition of cyclobutane, C₄H₈, to ethylene, C₂H₄, is represented by the following chemical equation:

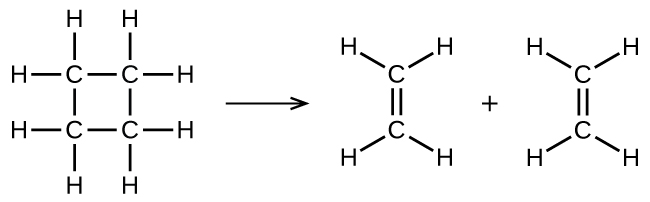
This equation represents the overall reaction observed, and it might also represent a legitimate unimolecular elementary reaction. The rate law predicted from this equation, assuming it is an elementary reaction, turns out to be the same as the rate law derived experimentally for the overall reaction, namely, one showing first-order behavior:

This agreement between observed and predicted rate laws is interpreted to mean that the proposed unimolecular, single-step process is a reasonable mechanism for the butadiene reaction.

10.1.2 Bimolecular Elementary Reactions

A bimolecular reaction involves two reactant species, for example:

For the first type, in which the two reactant molecules are different, the rate law is first-order in A and first order in B (second-order overall):

For the second type, in which two identical molecules collide and react, the rate law is second order in A:

Some chemical reactions occur by mechanisms that consist of a single bimolecular elementary reaction. One example is the reaction of nitrogen dioxide with carbon monoxide:

(see Figure 10.1)

Figure 10.1

The probable mechanism for the reaction between NO₂ and CO to yield NO and CO₂.

Bimolecular elementary reactions may also be involved as steps in a multistep reaction mechanism. The reaction of atomic oxygen with ozone is the second step of the two-step ozone decomposition mechanism discussed earlier in this section:

10.1.3 Termolecular Elementary Reactions

An elementary termolecular reaction involves the simultaneous collision of three atoms, molecules, or ions. Termolecular elementary reactions are uncommon because the probability of three particles colliding simultaneously is less than one one-thousandth of the probability of two particles colliding. There are, however, a few established termolecular elementary reactions. The reaction of nitric oxide with oxygen appears to involve termolecular steps:

Likewise, the reaction of nitric oxide with chlorine appears to involve termolecular steps:

10.1.4 Relating Reaction Mechanisms to Rate Laws

It’s often the case that one step in a multistep reaction mechanism is significantly slower than the others. Because a reaction cannot proceed faster than its slowest step, this step will limit the rate at which the overall reaction occurs. The slowest step is therefore called the rate-limiting step (or rate-determining step) of the reaction Figure 10.2.

Figure 10.2

A cattle chute is a nonchemical example of a rate-determining step. Cattle can only be moved from one holding pen to another as quickly as one animal can make its way through the chute. (credit: Loren Kerns)

As described earlier, rate laws may be derived directly from the chemical equations for elementary reactions. This is not the case, however, for ordinary chemical reactions. The balanced equations most often encountered represent the overall change for some chemical system, and very often this is the result of some multistep reaction mechanisms. In every case, the rate law must be determined from experimental data and the reaction mechanism subsequently deduced from the rate law (and sometimes from other data). The reaction of NO₂ and CO provides an illustrative example:

For temperatures above 225 °C, the rate law has been found to be:

The reaction is first order with respect to NO₂ and first-order with respect to CO. This is consistent with a single-step bimolecular mechanism and it is possible that this is the mechanism for this reaction at high temperatures.

At temperatures below 225 °C, the reaction is described by a rate law that is second order with respect to NO₂:

This rate law is not consistent with the single-step mechanism, but is consistent with the following two-step mechanism:

The rate-determining (slower) step gives a rate law showing second-order dependence on the NO₂ concentration, and the sum of the two equations gives the net overall reaction.

In general, when the rate-determining (slower) step is the first step in a mechanism, the rate law for the overall reaction is the same as the rate law for this step. However, when the rate-determining step is preceded by a step involving a rapidly reversible reaction the rate law for the overall reaction may be more difficult to derive.

As discussed in several chapters of this text, a reversible reaction is at equilibrium when the rates of the forward and reverse processes are equal. Consider the reversible elementary reaction in which NO dimerizes to yield an intermediate species N₂O₂. When this reaction is at equilibrium:

This expression may be rearranged to express the concentration of the intermediate in terms of the reactant NO:

Since intermediate species concentrations are not used in formulating rate laws for overall reactions, this approach is sometimes necessary, as illustrated in the following example exercise.

10.2 Catalysis

10.2.1 Catalysts Do Not Affect Equilibrium

A catalyst can speed up the rate of a reaction. Though this increase in reaction rate may cause a system to reach equilibrium more quickly (by speeding up the forward and reverse reactions), a catalyst has no effect on the value of an equilibrium constant nor on equilibrium concentrations.

The interplay of changes in concentration or pressure, temperature, and the lack of an influence of a catalyst on a chemical equilibrium is illustrated in the industrial synthesis of ammonia from nitrogen and hydrogen according to the equation

A large quantity of ammonia is manufactured by this reaction. Each year, ammonia is among the top 10 chemicals, by mass, manufactured in the world. About 2 billion pounds are manufactured in the United States each year.

Ammonia plays a vital role in our global economy. It is used in the production of fertilizers and is, itself, an important fertilizer for the growth of corn, cotton, and other crops. Large quantities of ammonia are converted to nitric acid, which plays an important role in the production of fertilizers, explosives, plastics, dyes, and fibers, and is also used in the steel industry.

10.2.2 PORTRAIT OF A CHEMIST

Fritz Haber

In the early 20th century, German chemist Fritz Haber (Figure 10.3) developed a practical process for converting diatomic nitrogen, which cannot be used by plants as a nutrient, to ammonia, a form of nitrogen that is easiest for plants to absorb.

$$\begin{array}{}\\ {\text{O}}_3⟶{\text{O}}_2+\text{O}\\ \text{O}+{\text{O}}_3⟶2{\text{O}}_2\end{array}$$

The availability of nitrogen is a strong limiting factor to the growth of plants. Despite accounting for 78% of air, diatomic nitrogen (N₂) is nutritionally unavailable due the tremendous stability of the nitrogen-nitrogen triple bond. For plants to use atmospheric nitrogen, the nitrogen must be converted to a more bioavailable form (this conversion is called nitrogen fixation).

Haber was born in Breslau, Prussia (presently Wroclaw, Poland) in December 1868. He went on to study chemistry and, while at the University of Karlsruhe, he developed what would later be known as the Haber process: the catalytic formation of ammonia from hydrogen and atmospheric nitrogen under high temperatures and pressures. For this work, Haber was awarded the 1918 Nobel Prize in Chemistry for synthesis of ammonia from its elements. The Haber process was a boon to agriculture, as it allowed the production of fertilizers to no longer be dependent on mined feed stocks such as sodium nitrate. Currently, the annual production of synthetic nitrogen fertilizers exceeds 100 million tons and synthetic fertilizer production has increased the number of humans that arable land can support from 1.9 persons per hectare in 1908 to 4.3 in 2008.

Figure 10.3

The work of Nobel Prize recipient Fritz Haber revolutionized agricultural practices in the early 20th century. His work also affected wartime strategies, adding chemical weapons to the artillery.

In addition to his work in ammonia production, Haber is also remembered by history as one of the fathers of chemical warfare. During World War I, he played a major role in the development of poisonous gases used for trench warfare. Regarding his role in these developments, Haber said, “During peace time a scientist belongs to the World, but during war time he belongs to his country.”¹ Haber defended the use of gas warfare against accusations that it was inhumane, saying that death was death, by whatever means it was inflicted. He stands as an example of the ethical dilemmas that face scientists in times of war and the double-edged nature of the sword of science.

Like Haber, the products made from ammonia can be multifaceted. In addition to their value for agriculture, nitrogen compounds can also be used to achieve destructive ends. Ammonium nitrate has also been used in explosives, including improvised explosive devices. Ammonium nitrate was one of the components of the bomb used in the attack on the Alfred P. Murrah Federal Building in downtown Oklahoma City on April 19, 1995.

It has long been known that nitrogen and hydrogen react to form ammonia. However, it became possible to manufacture ammonia in useful quantities by the reaction of nitrogen and hydrogen only in the early 20th century after the factors that influence its equilibrium were understood.

To be practical, an industrial process must give a large yield of product relatively quickly. One way to increase the yield of ammonia is to increase the pressure on the system in which N₂, H₂, and NH₃ are at equilibrium or are coming to equilibrium.

The formation of additional amounts of ammonia reduces the total pressure exerted by the system and somewhat reduces the stress of the increased pressure.

Although increasing the pressure of a mixture of N₂, H₂, and NH₃ will increase the yield of ammonia, at low temperatures, the rate of formation of ammonia is slow. At room temperature, for example, the reaction is so slow that if we prepared a mixture of N₂ and H₂, no detectable amount of ammonia would form during our lifetime. The formation of ammonia from hydrogen and nitrogen is an exothermic process:

Thus, increasing the temperature to increase the rate lowers the yield. If we lower the temperature to shift the equilibrium to favor the formation of more ammonia, equilibrium is reached more slowly because of the large decrease of reaction rate with decreasing temperature.

Part of the rate of formation lost by operating at lower temperatures can be recovered by using a catalyst. The net effect of the catalyst on the reaction is to cause equilibrium to be reached more rapidly.

In the commercial production of ammonia, conditions of about 500 °C, 150–900 atm, and the presence of a catalyst are used to give the best compromise among rate, yield, and the cost of the equipment necessary to produce and contain high-pressure gases at high temperatures (Figure 10.4).

Figure 10.4

Commercial production of ammonia requires heavy equipment to handle the high temperatures and pressures required. This schematic outlines the design of an ammonia plant.

Among the factors affecting chemical reaction rates discussed earlier in this chapter was the presence of a catalyst, a substance that can increase the reaction rate without being consumed in the reaction. The concepts introduced in the previous section on reaction mechanisms provide the basis for understanding how catalysts are able to accomplish this very important function.

Figure 10.5 shows reaction diagrams for a chemical process in the absence and presence of a catalyst. Inspection of the diagrams reveals several traits of these reactions. Consistent with the fact that the two diagrams represent the same overall reaction, both curves begin and end at the same energies (in this case, because products are more energetic than reactants, the reaction is endothermic). The reaction mechanisms, however, are clearly different. The uncatalyzed reaction proceeds via a one-step mechanism (one transition state observed), whereas the catalyzed reaction follows a two-step mechanism (two transition states observed) with a notably lesser activation energy. This difference illustrates the means by which a catalyst functions to accelerate reactions, namely, by providing an alternative reaction mechanism with a lower activation energy. Although the catalyzed reaction mechanism for a reaction needn’t necessarily involve a different number of steps than the uncatalyzed mechanism, it must provide a reaction path whose rate determining step is faster (lower E_a).

Figure 10.5

Reaction diagrams for an endothermic process in the absence (red curve) and presence (blue curve) of a catalyst. The catalyzed pathway involves a two-step mechanism (note the presence of two transition states) and an intermediate species (represented by the valley between the two transitions states).

EXAMPLE 10.2.3

Reaction Diagrams for Catalyzed Reactions

The two reaction diagrams here represent the same reaction: one without a catalyst and one with a catalyst. Estimate the activation energy for each process, and identify which one involves a catalyst.

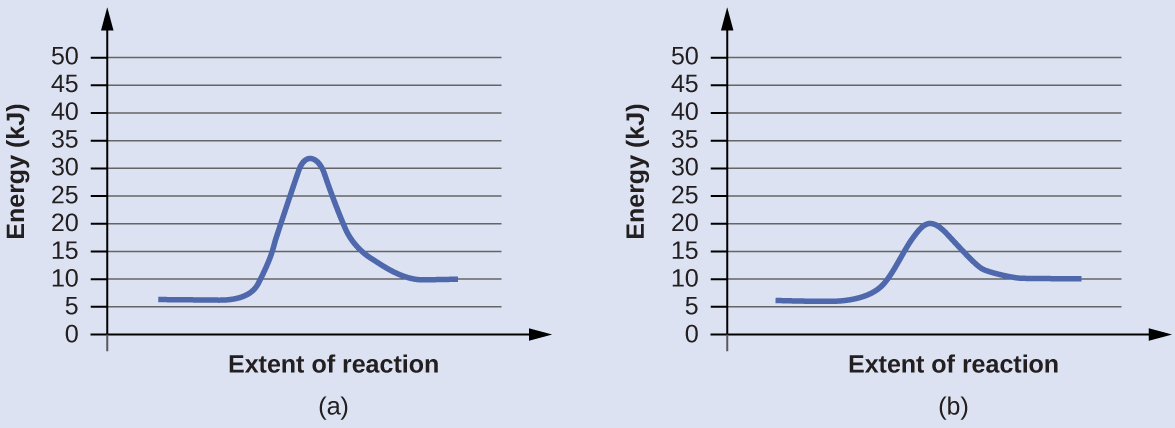
Solution

Activation energies are calculated by subtracting the reactant energy from the transition state energy.

$$\text{Rate}\left(r\right)=k\left(r\right)\left[B\right]$$

$$\frac{k\left(f\right)}{k\left(r\right)}=\frac{\left[B\right]}{\left[A\right]}$$

The catalyzed reaction is the one with lesser activation energy, in this case represented by diagram (b).

Check Your Learning

Reaction diagrams for a chemical process with and without a catalyst are shown below. Both reactions involve a two-step mechanism with a rate-determining first step. Compute activation energies for the first step of each mechanism, and identify which corresponds to the catalyzed reaction. How do the second steps of these two mechanisms compare?

Answer

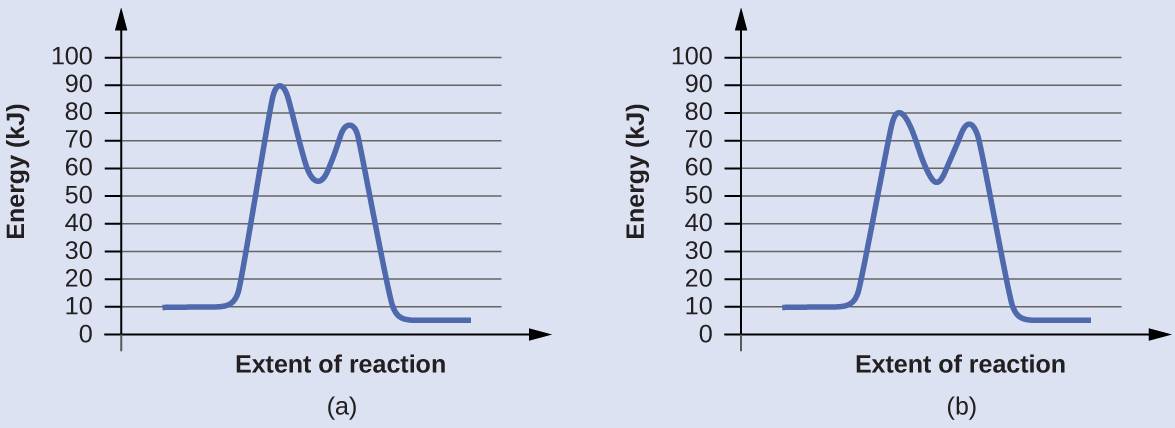
For the first step, E_a = 80 kJ for (a) and 70 kJ for (b), so diagram (b) depicts the catalyzed reaction. Activation energies for the second steps of both mechanisms are the same, 20 kJ.

10.2.4 Homogeneous Catalysts

A homogeneous catalyst is present in the same phase as the reactants. It interacts with a reactant to form an intermediate substance, which then decomposes or reacts with another reactant in one or more steps to regenerate the original catalyst and form product.

As an important illustration of homogeneous catalysis, consider the earth’s ozone layer. Ozone in the upper atmosphere, which protects the earth from ultraviolet radiation, is formed when oxygen molecules absorb ultraviolet light and undergo the reaction:

Ozone is a relatively unstable molecule that decomposes to yield diatomic oxygen by the reverse of this equation. This decomposition reaction is consistent with the following two-step mechanism:

A number of substances can catalyze the decomposition of ozone. For example, the nitric oxide -catalyzed decomposition of ozone is believed to occur via the following three-step mechanism:

As required, the overall reaction is the same for both the two-step uncatalyzed mechanism and the three-step NO-catalyzed mechanism:

Notice that NO is a reactant in the first step of the mechanism and a product in the last step. This is another characteristic trait of a catalyst: Though it participates in the chemical reaction, it is not consumed by the reaction.

10.2.5 PORTRAIT OF A CHEMIST

Mario J. Molina

The 1995 Nobel Prize in Chemistry was shared by Paul J. Crutzen, Mario J. Molina (Figure 10.6), and F. Sherwood Rowland “for their work in atmospheric chemistry, particularly concerning the formation and decomposition of ozone.”² Molina, a Mexican citizen, carried out the majority of his work at the Massachusetts Institute of Technology (MIT).

Figure 10.6

(a) Mexican chemist Mario Molina (1943 –) shared the Nobel Prize in Chemistry in 1995 for his research on (b) the Antarctic ozone hole. (credit a: courtesy of Mario Molina; credit b: modification of work by NASA)

In 1974, Molina and Rowland published a paper in the journal Nature detailing the threat of chlorofluorocarbon gases to the stability of the ozone layer in earth’s upper atmosphere. The ozone layer protects earth from solar radiation by absorbing ultraviolet light. As chemical reactions deplete the amount of ozone in the upper atmosphere, a measurable “hole” forms above Antarctica, and an increase in the amount of solar ultraviolet radiation— strongly linked to the prevalence of skin cancers—reaches earth’s surface. The work of Molina and Rowland was instrumental in the adoption of the Montreal Protocol, an international treaty signed in 1987 that successfully began phasing out production of chemicals linked to ozone destruction.

Molina and Rowland demonstrated that chlorine atoms from human-made chemicals can catalyze ozone destruction in a process similar to that by which NO accelerates the depletion of ozone. Chlorine atoms are generated when chlorocarbons or chlorofluorocarbons—once widely used as refrigerants and propellants—are photochemically decomposed by ultraviolet light or react with hydroxyl radicals. A sample mechanism is shown here using methyl chloride:

$${\text{CH}}_3\text{Cl}+\text{OH}⟶\text{Cl}+\text{other products}$$

Chlorine radicals break down ozone and are regenerated by the following catalytic cycle:

$$\begin{array}{}\\ \text{Cl}+{\text{O}}_3⟶\text{ClO}+{\text{O}}_2\\ \text{ClO}+\text{O}⟶\text{Cl}+{\text{O}}_2\\ \text{overall Reaction:}{\text{O}}_3+\text{O}⟶2{\text{O}}_2\end{array}$$

A single monatomic chlorine can break down thousands of ozone molecules. Luckily, the majority of atmospheric chlorine exists as the catalytically inactive forms Cl₂ and ClONO₂.

Since receiving his portion of the Nobel Prize, Molina has continued his work in atmospheric chemistry at MIT.

10.2.6 HOW SCIENCES INTERCONNECT

Glucose-6-Phosphate Dehydrogenase Deficiency

Enzymes in the human body act as catalysts for important chemical reactions in cellular metabolism. As such, a deficiency of a particular enzyme can translate to a life-threatening disease. G6PD (glucose-6-phosphate dehydrogenase) deficiency, a genetic condition that results in a shortage of the enzyme glucose-6-phosphate dehydrogenase, is the most common enzyme deficiency in humans. This enzyme, shown in Figure 10.7, is the rate-limiting enzyme for the metabolic pathway that supplies NADPH to cells (Figure 10.8).

Figure 10.7

Glucose-6-phosphate dehydrogenase is a rate-limiting enzyme for the metabolic pathway that supplies NADPH to cells.

A disruption in this pathway can lead to reduced glutathione in red blood cells; once all glutathione is consumed, enzymes and other proteins such as hemoglobin are susceptible to damage. For example, hemoglobin can be metabolized to bilirubin, which leads to jaundice, a condition that can become severe. People who suffer from G6PD deficiency must avoid certain foods and medicines containing chemicals that can trigger damage their glutathione-deficient red blood cells.

Figure 10.8

In the mechanism for the pentose phosphate pathway, G6PD catalyzes the reaction that regulates NADPH, a co-enzyme that regulates glutathione, an antioxidant that protects red blood cells and other cells from oxidative damage.

10.2.7 Heterogeneous Catalysts

A heterogeneous catalyst is a catalyst that is present in a different phase (usually a solid) than the reactants. Such catalysts generally function by furnishing an active surface upon which a reaction can occur. Gas and liquid phase reactions catalyzed by heterogeneous catalysts occur on the surface of the catalyst rather than within the gas or liquid phase.

Heterogeneous catalysis typically involves the following processes:

1. Adsorption of the reactant(s) onto the surface of the catalyst
2. Activation of the adsorbed reactant(s)
3. Reaction of the adsorbed reactant(s)
4. Desorption of product(s) from the surface of the catalyst

Figure 10.9 illustrates the steps of a mechanism for the reaction of compounds containing a carbon–carbon double bond with hydrogen on a nickel catalyst. Nickel is the catalyst used in the hydrogenation of polyunsaturated fats and oils (which contain several carbon–carbon double bonds) to produce saturated fats and oils (which contain only carbon–carbon single bonds).

Figure 10.9

Mechanism for the Ni-catalyzed reaction ${\text{C}}_2{\text{H}}_4+{\text{H}}_2⟶{\text{C}}_2{\text{H}}_6.$ (a) Hydrogen is adsorbed on the surface, breaking the H–H bonds and forming Ni–H bonds. (b) Ethylene is adsorbed on the surface, breaking the C-C π-bond and forming Ni–C bonds. (c) Atoms diffuse across the surface and form new C–H bonds when they collide. (d) C₂H₆ molecules desorb from the Ni surface.

Many important chemical products are prepared via industrial processes that use heterogeneous catalysts, including ammonia, nitric acid, sulfuric acid, and methanol. Heterogeneous catalysts are also used in the catalytic converters found on most gasoline-powered automobiles (Figure 10.10).

10.2.8 CHEMISTRY IN EVERYDAY LIFE

Automobile Catalytic Converters

Scientists developed catalytic converters to reduce the amount of toxic emissions produced by burning gasoline in internal combustion engines. By utilizing a carefully selected blend of catalytically active metals, it is possible to effect complete combustion of all carbon-containing compounds to carbon dioxide while also reducing the output of nitrogen oxides. This is particularly impressive when we consider that one step involves adding more oxygen to the molecule and the other involves removing the oxygen (Figure 10.10).

Figure 10.10

A catalytic converter allows for the combustion of all carbon-containing compounds to carbon dioxide, while at the same time reducing the output of nitrogen oxide and other pollutants in emissions from gasoline-burning engines.

Most modern, three-way catalytic converters possess a surface impregnated with a platinum-rhodium catalyst, which catalyzes the conversion of nitric oxide into dinitrogen and oxygen as well as the conversion of carbon monoxide and hydrocarbons such as octane into carbon dioxide and water vapor:

$$\begin{array}{l}2{\text{NO}}_{\text{2}}(g)⟶{\text{N}}_{\text{2}}(g)+2{\text{O}}_{\text{2}}(g)\\ 2\text{CO(}g)+{\text{O}}_2(g)⟶2{\text{CO}}_{\text{2}}(g)\\ 2{\text{C}}_8{\text{H}}_{18}(g)+25{\text{O}}_2(g)⟶16{\text{CO}}_2(g)+18{\text{H}}_2\text{O}(g)\end{array}$$

In order to be as efficient as possible, most catalytic converters are preheated by an electric heater. This ensures that the metals in the catalyst are fully active even before the automobile exhaust is hot enough to maintain appropriate reaction temperatures.

10.2.9 HOW SCIENCES INTERCONNECT

Enzyme Structure and Function

The study of enzymes is an important interconnection between biology and chemistry. Enzymes are usually proteins (polypeptides) that help to control the rate of chemical reactions between biologically important compounds, particularly those that are involved in cellular metabolism. Different classes of enzymes perform a variety of functions, as shown in Table 10.1.

Table 10.11

Classes of Enzymes and Their Functions

Class	Function
oxidoreductases	redox reactions
transferases	transfer of functional groups
hydrolases	hydrolysis reactions
lyases	group elimination to form double bonds
isomerases	isomerization
ligases	bond formation with ATP hydrolysis

Enzyme molecules possess an active site, a part of the molecule with a shape that allows it to bond to a specific substrate (a reactant molecule), forming an enzyme-substrate complex as a reaction intermediate. There are two models that attempt to explain how this active site works. The most simplistic model is referred to as the lock-and-key hypothesis, which suggests that the molecular shapes of the active site and substrate are complementary, fitting together like a key in a lock. The induced fit hypothesis, on the other hand, suggests that the enzyme molecule is flexible and changes shape to accommodate a bond with the substrate. This is not to suggest that an enzyme’s active site is completely malleable, however. Both the lock-and-key model and the induced fit model account for the fact that enzymes can only bind with specific substrates, since in general a particular enzyme only catalyzes a particular reaction (Figure 10.11).

Figure 10.12

(a) According to the lock-and-key model, the shape of an enzyme’s active site is a perfect fit for the substrate. (b) According to the induced fit model, the active site is somewhat flexible, and can change shape in order to bond with the substrate.

The connection between the rate of a reaction and its equilibrium constant is one we can easily determine with just a bit of algebraic substitution. For a reaction where substance A forms B (and the reverse)

The rate of the forward reaction is

And the rate of the reverse reaction is

Once equilibrium is established, the rates of the forward and reverse reactions are equal:

Rearranging a bit, we get

Also recall that the equilibrium constant is simply the ratio of product to reactant concentration at equilibrium:

So the equilibrium constant turns out to be the ratio of the forward to the reverse rate constants. This relationship also helps cement our understanding of the nature of a catalyst. That is, a catalyst does not change the fundamental equilibrium (or the underlying thermodynamics) of a reaction. Rather, what it does is alter the rate constant for the reaction – that is, both rate constants, forward and reverse, equally. In doing so, catalysts usually speed up the rate at which reactions attain equilibrium (though they can be used to slow down the rate of reaction as well!).